There are literally dozens of proofs for the Pythagorean Theorem. The proof shown here is probably the clearest and easiest to understand. The Pythagorean Theorem states that for any right triangle the square of the hypotenuse equals the sum of the squares of the other 2 sides. If we draw a right triangle having sides 'a' 'b' and 'c' (with 'c' being the hypotenuse) then according to the theorem, the length of c² = a² + b² In order to prove the theorem, we construct squares on each of the sides of the triangle. It is important to realize that squaring the length of side a is exactly the same thing as determining the area of the green square. (The same applies to side b with the red square and side c with the blue square and this is the important concept of this proof). Basically, if we can show that the area of the green square plus the area of the red square equals the area of the blue square, we have proven the Pythagorean Theorem. 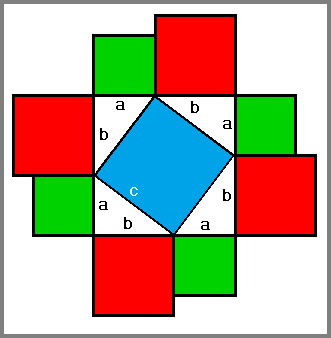Now let's construct those same squares around the remaining three sides of the blue square. Gee, that diagram sure looks confusing doesn't it? However, you can see that those eight squares have "drawn" a square around the blue square. When we remove the 6 squares we just added, we'll have a diagram very similar to the first one except now the blue square is surrounded by a square with sides of a length 'a' plus 'b'. The area of this new square would be (a + b)² and the new diagram would look like the one drawn below. 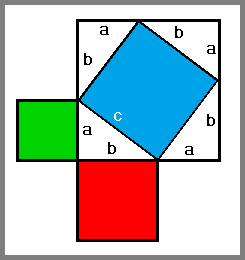Area of green square = a²   Area of red square = b² The area of the larger square surrounding the blue square equals (a+b)² which equals a² + 2ab + b². Note that the blue square is surrounded by 4 right triangles, the area of each being ½ (a•b) making the total area of all 4 triangles equal 2•a•b. So, the area of the blue square = area of the surrounding square minus the area of the 4 triangles. Area of blue square = a² + 2ab + b² minus 2ab Blue Square Area = c² = a² + b² We have just proven the Pythagorean Theorem.